The project is entitled "Combinatorial rigidity, symmetric geometric constraint systems, and applications" and concerns the rigidity and flexibility of discrete structures which are defined by geometric constraints, such as fixed lengths or fixed directions, on a set of rigid objects, such as points or lines. This topic is a particular specialism of the department, with four academic staff and two PhD students in the Discrete Mathematics and Geometric Rigidity research group.

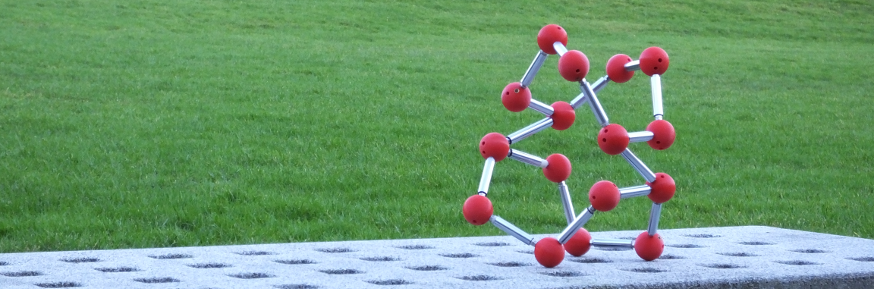
The main objective is to investigate the impact of symmetry on the rigidity and flexibility properties of such structures, and in particular to obtain combinatorial characterisations of rigid structures which are as generic as possible subject to the given symmetry constraints. An example is shown in the picture above, a framework model that is flexible because it has dihedral symmetry.

Since symmetry is ubiquitous in both natural and man-made structures, the potential for applications of these results is considerable. In particular, the project aims to design new algorithms for the rigidity analysis of symmetric proteins, as well as to develop new architectures and faster algorithms for the control of multi-robot formations. More details may be found via the above link.

The project will begin in Summer 2015 and will fund a Research Associate position in the department.