
Pranav Acharya
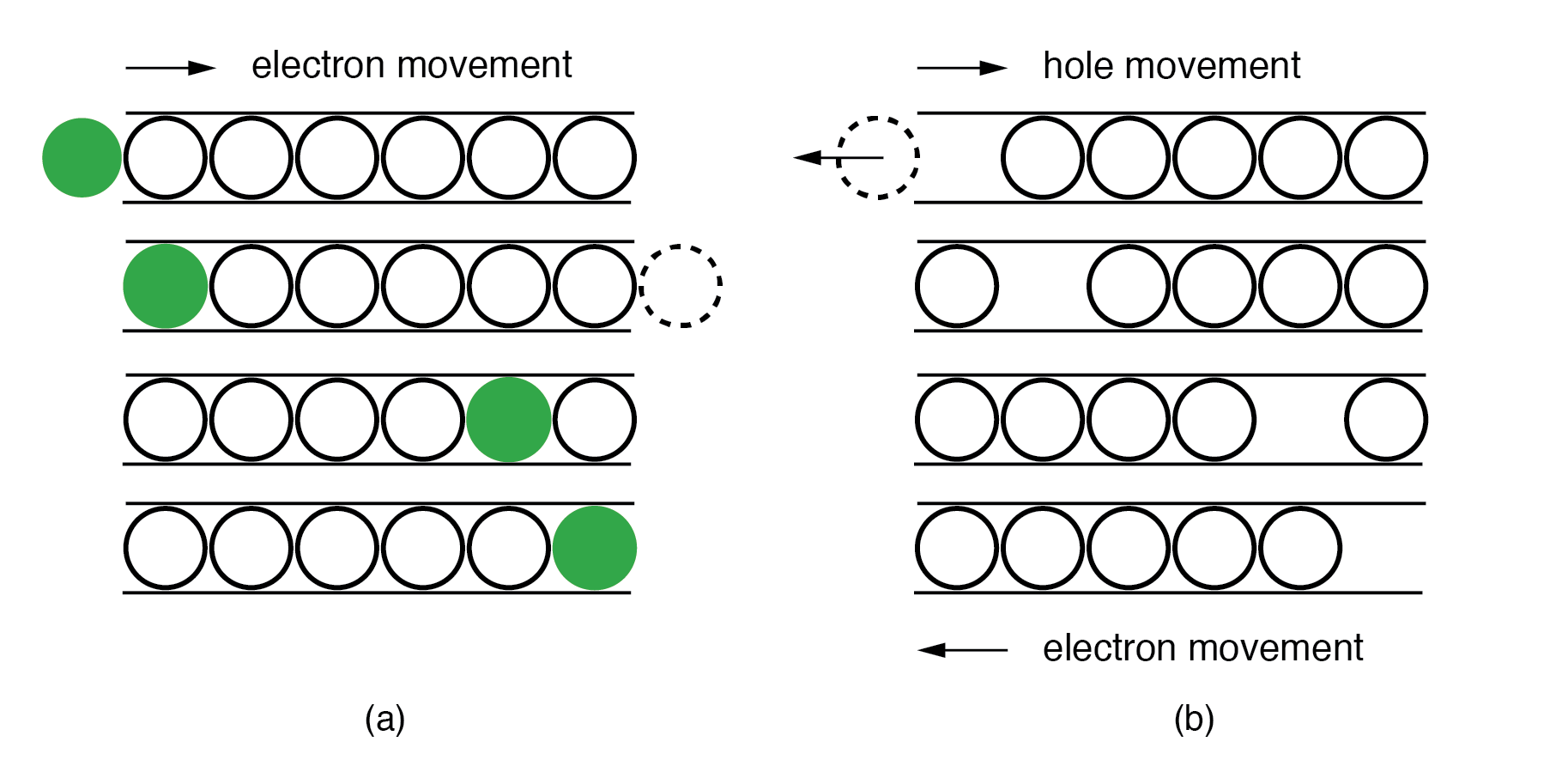
Photons create electron-hole pairs in semiconductors, and negatively charged electrons and positively charged holes interact and bind with each other to form excitonic complexes.
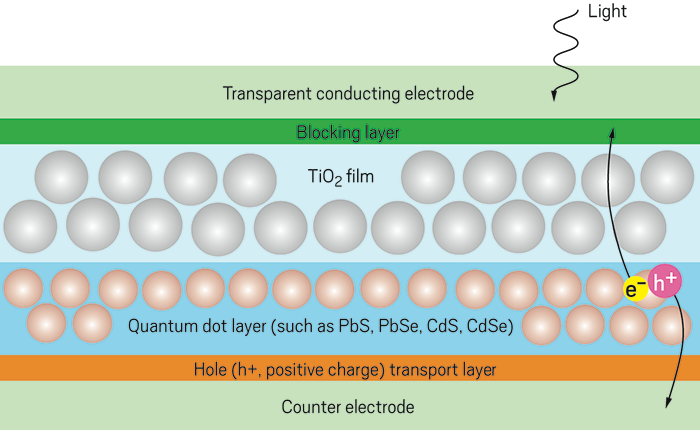
Quantum dots are nanometre-scale semiconductor particles, which have a wide variety of applications such as high efficiency photovoltaics, for solar panels, or quantum computers.
I am simulating excitonic complexes in quantum dots to calculate their binding energies, charge densities, and distribution in quantum dots. This research is important because these properties of excitonic complexes relate to the photoluminescence spectra and optoelectronic properties of quantum dots. My research will focus on type 2 quantum dots, which contain only one type of charge carrier, which in my case will have holes inside and electrons outside. The simulations will be carried out using Quantum Monte Carlo, a computational framework to simulate solids while taking into account quantum effects of atoms, electrons and holes. I am researching this due to an interest in what happens at small scales in solids, and because this research will have an impact in industry, by helping future modelling and optimisation of quantum dots for applications.

Pranav Acharya
![QLED Samsung TV[2]](https://www.lancaster.ac.uk/2021ugconference/wp-content/uploads/lica-files/e2tb3mxn/uk-qledtv-q85t-qe75q85tatxxu-titanumsilver-235584816.png)
Dr Neil Drummond is my supervisor for this project, and has taught me the skills necessary for this project and provided guidance throughout it.































































































































































































































































































































































































































































